Parallel and Perpendicular Lines, Transversals, Alternate Interior Angles, Alternate Exterior Angles
The Organic Chemistry Tutor・24 minutes read
Parallel lines share the same slope and do not intersect, while perpendicular lines intersect at a right angle; various types of angles formed by transversals with parallel lines include congruent alternate interior and exterior angles, as well as supplementary consecutive interior angles. The text also emphasizes the sum of interior angles in triangles and quadrilaterals, providing examples to illustrate the relationships between complementary and supplementary angles.
Insights
- Parallel lines, such as line A and line B, maintain the same slope and do not intersect, which means that if one line has a slope of 3/4, the other will also have a slope of 3/4; this fundamental property is crucial in understanding the behavior of parallel lines in geometry.
- The text emphasizes the relationships between different angles formed by intersecting lines, highlighting that vertical angles are congruent, alternate interior angles are equal, and consecutive interior angles are supplementary, which provides a framework for solving various geometric problems involving angles and their relationships.
Get key ideas from YouTube videos. It’s free
Recent questions
What are complementary angles?
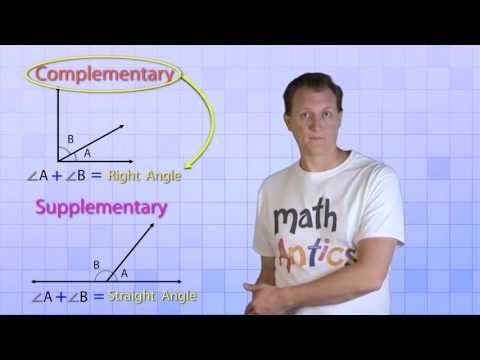
Complementary angles are two angles that add up to 90 degrees. For example, if one angle measures 30 degrees, the other must measure 60 degrees to complete the total of 90 degrees. This relationship is often used in various geometric problems, particularly in right triangles, where one angle is always 90 degrees. Understanding complementary angles is essential for solving problems involving angle relationships, especially in trigonometry and geometry, where these angles frequently appear in calculations and proofs.
How do you find the slope of a line?
The slope of a line is determined by the ratio of the vertical change to the horizontal change between two points on the line. This is often expressed as "rise over run." For example, if you have two points, (x1, y1) and (x2, y2), the slope (m) can be calculated using the formula m = (y2 - y1) / (x2 - x1). A positive slope indicates that the line rises as it moves from left to right, while a negative slope indicates a decline. Understanding how to calculate the slope is fundamental in algebra and geometry, as it helps in graphing linear equations and analyzing relationships between variables.
What are vertical angles?
Vertical angles are the angles that are opposite each other when two lines intersect. They are formed by the intersection of two lines and are always congruent, meaning they have the same measure. For instance, if two lines intersect and one of the angles measures 50 degrees, the angle directly across from it will also measure 50 degrees. This property of vertical angles is a key concept in geometry, as it helps in solving various problems related to angle relationships and is often used in proofs and theorems involving intersecting lines.
What is a transversal line?
A transversal line is a line that intersects two or more other lines at distinct points. When a transversal crosses parallel lines, it creates several angles, including interior and exterior angles. For example, if line C is a transversal that intersects lines A and B, it forms angles that can be classified as corresponding, alternate interior, and consecutive interior angles. Understanding transversals is crucial in geometry, as it helps in analyzing angle relationships and solving problems related to parallel lines and angles formed by their intersection.
How do you calculate the sum of angles in a triangle?
The sum of the interior angles in a triangle is always 180 degrees, regardless of the type of triangle. This fundamental property can be used to find missing angles when the measures of the other two angles are known. For example, if one angle measures 40 degrees and another measures 50 degrees, the third angle can be calculated by subtracting the sum of the known angles from 180 degrees: 180 - (40 + 50) = 90 degrees. This principle is essential in various geometric applications, including proofs, constructions, and solving real-world problems involving triangles.
Related videos

BYJU'S - Class 9 & 10
Lines and Angles Class 9 Maths in One Shot (Solving Important Questions/MCQs) | BYJU'S Class 9
mathantics
Math Antics - Angle Basics

Class 9 Learn With Mansi
Lines And Angles | Exercise 6.1 | Chapter 6 | SEED 2024-2025

The Organic Chemistry Tutor
Introduction to Geometry

BYJU'S
Understanding Quadrilaterals | Learn with BYJU'S
Summary
00:00
Understanding Lines and Angles in Geometry
- Parallel lines, such as line A and line B, do not intersect and share the same slope; for example, if line A has a slope of 3/4, line B also has a slope of 3/4.
- Perpendicular lines intersect at a right angle (90°); for instance, if line A is perpendicular to line B, they meet at this angle.
- To find the slope of a line perpendicular to another, flip the fraction and change the sign; if line C has a slope of 3/4, the slope of line D will be -4/3.
- A transversal line, such as line C, intersects two parallel lines (A and B), creating angles; angles 3, 4, 5, and 6 are interior angles, while angles 1, 2, 7, and 8 are exterior angles.
- Alternate interior angles, like angles 3 and 6, are congruent and located on opposite sides of the transversal; similarly, angles 4 and 5 are also alternate interior angles and congruent.
- Alternate exterior angles, such as angles 1 and 8, and angles 2 and 7, are also congruent and located outside the parallel lines, alternating with respect to the transversal.
- Corresponding angles, like angles 2 and 6, are congruent and occupy the same relative position at each intersection of the transversal with the parallel lines.
- Consecutive interior angles, such as angles 4 and 6, are supplementary, meaning they add up to 180°; for example, angle 4 + angle 6 = 180°.
- Vertical angles, formed when two lines intersect, are congruent; for instance, if angle 1 is 50°, angle 4 is also 50°, and angles 2 and 3 are congruent as well.
- Complementary angles add up to 90°; for example, if angle C is 30°, then angle A must be 60° since 90° - 30° = 60°, while supplementary angles add up to 180°, such as angle ABD (40°) and angle DBC (140°) totaling 180°.
23:30
Understanding Angles and Their Relationships
- The initial angle is 40 degrees, leading to the identification of vertical angles, where the opposite angle is also 40 degrees, and the supplementary angle is calculated as 180 - 40 = 140 degrees.
- The next angle is determined to be 50 degrees, which is also a vertical angle, and the supplementary angle is found by 180 - 50 = 130 degrees, resulting in another angle of 130 degrees.
- The angles are classified as consecutive interior angles, which sum to 180 degrees, confirming that one angle is 130 degrees, while alternate interior angles are identified as 50 degrees, leading to congruent alternate exterior angles of 130 degrees.
- The problem introduces angle X, which is part of a triangle where the sum of angles must equal 180 degrees; thus, 40 + 50 + X = 180, resulting in X = 90 degrees.
- A new scenario presents an angle of 60 degrees and another of 110 degrees, leading to supplementary angles of 70 degrees each, and the missing angle X is calculated as 60 + 70 + X = 180, giving X = 50 degrees.
- The text explains that the sum of interior angles in a triangle is always 180 degrees, while for a quadrilateral, the sum is 360 degrees, derived from the formula (n - 2) * 180, where n is the number of sides.
- For a pentagon, the sum of the interior angles is calculated as (5 - 2) * 180 = 540 degrees, indicating that the sum of all five angles must equal 540 degrees.
- Several word problems are presented, including finding angle B when angle A is 32 degrees (B = 90 - 32 = 58 degrees), and determining angle A when angle B is 74 degrees (A = 180 - 74 = 106 degrees), demonstrating the application of complementary and supplementary angle relationships.




