Factoring completely with a common factor | Algebra 1 | Khan Academy
Khan Academy・4 minutes read
To factor expressions, the greatest common factor (GCF) must first be identified and extracted, allowing further simplification into products of binomials, as shown in the examples of \(4(x - 3)(x + 1)\) and \(-3(x - 5)(x - 2)\). A systematic approach involves finding two numbers that meet specific addition and multiplication criteria based on the quadratic coefficients, ensuring accurate factorizations.
Insights
- Identifying the greatest common factor (GCF) is crucial in factoring expressions, as it simplifies the process significantly; for instance, in the expression \(4x^2 8x - 12\), factoring out the GCF of 4 leads to a simpler quadratic \(4(x^2 - 2x - 3)\), which can be further broken down into \(4(x - 3)(x + 1)\).
- When dealing with quadratic expressions, it is important to find two specific numbers that meet both the addition and multiplication criteria based on the quadratic's coefficients; in the example of \(-3x^2 + 21x 30\), the numbers \(-5\) and \(-2\) were crucial in achieving the complete factorization of \(-3(x - 5)(x - 2)\), illustrating a systematic method for solving such problems.
Get key ideas from YouTube videos. It’s free
Recent questions
What is the greatest common factor?
The greatest common factor (GCF) is the largest integer that divides all the terms in a given expression without leaving a remainder. It is a crucial concept in mathematics, particularly in simplifying expressions and factoring polynomials. To find the GCF, one must identify the common factors of each term and select the highest one. For example, in the expression \(4x^2 - 8x - 12\), the GCF is 4, as it is the largest number that can evenly divide each coefficient in the expression. Recognizing the GCF allows for easier manipulation of algebraic expressions and is often the first step in the factoring process.
How do you factor a quadratic expression?
Factoring a quadratic expression involves rewriting it as a product of two binomials. The process typically starts by identifying the coefficients of the quadratic, which is in the standard form \(ax^2 + bx + c\). The goal is to find two numbers that multiply to \(ac\) (the product of the coefficient of \(x^2\) and the constant term) and add to \(b\) (the coefficient of \(x\)). For instance, in the quadratic \(x^2 - 7x + 10\), one would look for two numbers that multiply to 10 and add to -7. The numbers -5 and -2 satisfy these conditions, allowing the expression to be factored as \((x - 5)(x - 2)\). This method is essential for solving quadratic equations and simplifying expressions.
What does it mean to factor an expression?
Factoring an expression means breaking it down into simpler components, typically as a product of its factors. This process is fundamental in algebra, as it allows for easier manipulation and solving of equations. When an expression is factored, it is expressed in a form that reveals its roots or solutions. For example, factoring the expression \(4x^2 - 8x - 12\) involves identifying the greatest common factor and rewriting it as \(4(x^2 - 2x - 3)\), which can be further factored into \(4(x - 3)(x + 1)\). This simplification not only makes calculations easier but also provides insights into the behavior of the function represented by the expression.
Why is factoring important in algebra?
Factoring is a critical skill in algebra because it simplifies complex expressions and enables the solving of equations. By breaking down polynomials into their factors, one can easily identify the roots of the equation, which are the values of the variable that make the equation true. This is particularly useful in quadratic equations, where factoring can lead to solutions that are otherwise difficult to find. Additionally, factoring helps in simplifying fractions and performing polynomial long division. Overall, mastering factoring techniques enhances problem-solving abilities and deepens understanding of algebraic concepts.
How do you find factors of a polynomial?
To find the factors of a polynomial, one typically starts by identifying the greatest common factor (GCF) of all the terms in the polynomial. Once the GCF is factored out, the remaining polynomial can often be further factored, especially if it is a quadratic. For example, in the polynomial \(-3x^2 + 21x - 30\), the GCF is \(-3\), which can be factored out to yield \(-3(x^2 - 7x + 10)\). The next step involves finding two numbers that multiply to the constant term (10) and add to the linear coefficient (-7). In this case, the numbers -5 and -2 work, allowing the polynomial to be completely factored as \(-3(x - 5)(x - 2)\). This systematic approach is essential for simplifying polynomials and solving equations.
Related videos
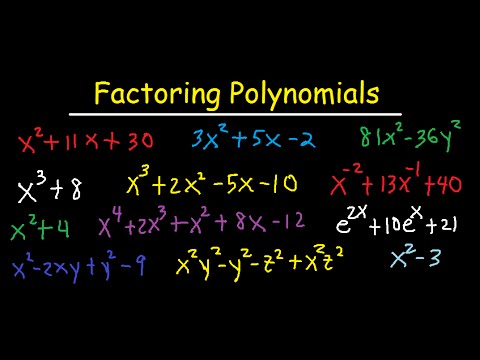
The Organic Chemistry Tutor
Factoring Polynomials - By GCF, AC Method, Grouping, Substitution, Sum & Difference of Cubes

Mrs Townsend Mathematics - South Africa
Grade 9 Mathematics - Factorisation Part 1
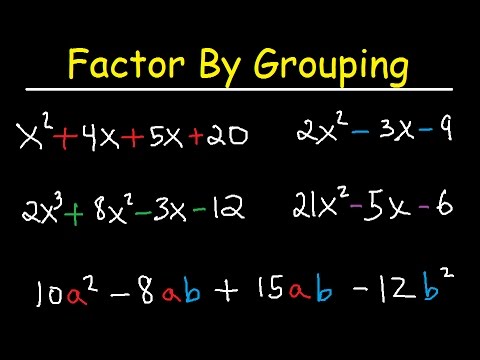
The Organic Chemistry Tutor
Factor By Grouping Polynomials - 4 Terms, Trinomials - 3 Terms, Algebra 2

The Organic Chemistry Tutor
How To Factor Polynomials The Easy Way!

jawiskunde
Ontbinden in factoren
Summary
00:00
Mastering Quadratic Factorization Techniques
- The first step in factoring an expression is to identify the greatest common factor (GCF) of all terms. In the example provided, the GCF is 4, which can be factored out from the expression \(4x^2 - 8x - 12\), resulting in \(4(x^2 - 2x - 3)\). This further factors into \(4(x - 3)(x + 1)\), completing the factorization.
- In the second example, the expression \(-3x^2 + 21x - 30\) is simplified by factoring out a negative GCF of \(-3\), yielding \(-3(x^2 - 7x + 10)\). This quadratic can be factored further by finding two numbers that add to \(-7\) and multiply to \(10\), which are \(-5\) and \(-2\). Thus, the complete factorization is \(-3(x - 5)(x - 2)\).
- When factoring quadratics, it is essential to find two numbers that satisfy both the addition and multiplication conditions based on the coefficients of the quadratic. For example, in the first case, the numbers \(-3\) and \(1\) were used, while in the second case, \(-5\) and \(-2\) were identified, demonstrating the systematic approach to factorization.




