Ontbinden in factoren
jawiskunde・2 minutes read
Factorization involves simplifying expressions by breaking them down into common factors and applying intermediate steps to form pairs of brackets. Recognizing remarkable products and differences of two squares also aids in decomposing expressions into pairs of brackets for further simplification.
Insights
- Factorization involves simplifying expressions by removing brackets using the arc method from right to left, breaking down into common factors, and applying intermediate steps for pair-wise bracket formation.
- Recognizing remarkable products and differences of two squares enables further decomposition into pairs of brackets, as shown with examples like x^2 - 4 and x^2 - 25, expanding the scope of factorization techniques.
Get key ideas from YouTube videos. It’s free
Recent questions
How is factorization achieved?
Factorization involves removing brackets using the arc method, working from right to left. By breaking down expressions into common factors and applying intermediate steps, one can simplify and factorize expressions into pairs of brackets.
What are remarkable products in factorization?
Remarkable products, like x squared - 2x + 2x - 4 simplifying to x k - 4, are key in factorization. Recognizing differences of two squares allows for further decomposition into pairs of brackets, as demonstrated with examples like x - 4 ma x + 4 and x - 5 times x + 5.
Why is the arc method used in factorization?
The arc method is used in factorization to remove brackets efficiently. Working from right to left, this method breaks down expressions into common factors and simplifies them into pairs of brackets through intermediate steps.
How does recognizing common factors aid in factorization?
Recognizing common factors aids in factorization by simplifying expressions into pairs of brackets. By identifying and breaking down these factors, one can efficiently factorize complex expressions.
What role do intermediate steps play in factorization?
Intermediate steps in factorization, like simplifying expressions into common factors, are crucial. By applying these steps, one can effectively break down and factorize expressions into pairs of brackets, leading to a simplified form.
Related videos

Mrs Townsend Mathematics - South Africa
Grade 9 Mathematics - Factorisation Part 1

Khan Academy
Factoring completely with a common factor | Algebra 1 | Khan Academy
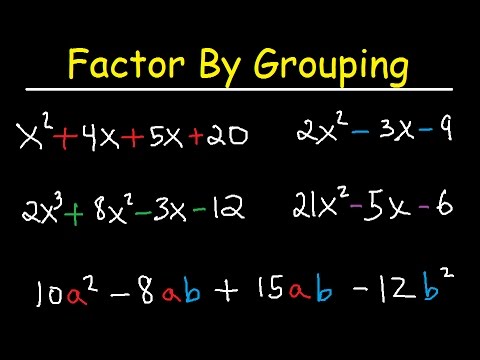
The Organic Chemistry Tutor
Factor By Grouping Polynomials - 4 Terms, Trinomials - 3 Terms, Algebra 2
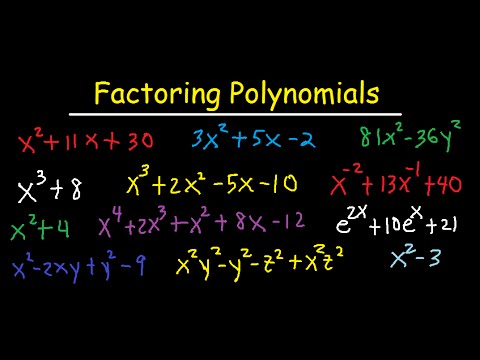
The Organic Chemistry Tutor
Factoring Polynomials - By GCF, AC Method, Grouping, Substitution, Sum & Difference of Cubes

Brian McLogan
Easy Medium Hard Simplify Complex Fractions
Summary
00:00
Simplify and Factorize Expressions with Brackets
- Factorization involves removing brackets using the arc method, working from right to left. By breaking down expressions into common factors, such as 3x to 4 - 6x, and applying intermediate steps, like 3x to 4, one can simplify and factorize expressions into pairs of brackets.
- Understanding remarkable products, like x squared - 2x + 2x - 4 simplifying to x k - 4, and recognizing differences of two squares, allows for further decomposition into pairs of brackets, as demonstrated with examples like x - 4 ma x + 4 and x - 5 times x + 5.




