Grade 9 Mathematics - Factorisation Part 1
Mrs Townsend Mathematics - South Africa・12 minutes read
Factorization is the process of taking the highest common factor from an expression, exemplified by simplifying 4x + 8 into 4(x + 2), while it also involves recognizing structures like the difference of two perfect squares, such as turning x^2 - 16 into (x - 4)(x + 4). To effectively factor expressions, one must identify both the numerical and variable components, ensuring proper use of brackets and understanding how to handle perfect squares and shared factors.
Insights
- Factorization is a process that reverses simplification by extracting the highest common factor (HCF) from an expression, as illustrated by factoring 4x + 8 into 4(x + 2), which highlights the importance of identifying both numerical and variable components in the terms being factored.
- The difference of two perfect squares can be effectively factorized using a specific format that involves two brackets, one with a minus sign and the other with a plus sign; for instance, expressions like x^2 16 and 9p^2 - 1 can be factored into (x - 4)(x + 4) and (3p - 1)(3p + 1), respectively, emphasizing a systematic approach to recognizing and applying this principle.
Get key ideas from YouTube videos. It’s free
Recent questions
What is factorization in mathematics?
Factorization in mathematics refers to the process of breaking down an expression into a product of its factors. This is essentially the reverse of simplification or distribution, where an expression is expanded. For example, if you have an expression like 4x + 8, factorization involves identifying the highest common factor (HCF) that can be extracted from the terms. In this case, the HCF is 4, allowing us to rewrite the expression as 4(x + 2). This process is crucial in algebra as it simplifies expressions and makes solving equations easier.
How do I find the highest common factor?
To find the highest common factor (HCF) of two terms, you need to identify the largest number and the most variables that can be factored out from both terms. For instance, consider the terms 6x and -9. The HCF here is 3, as it is the largest number that divides both coefficients. Additionally, since both terms share the variable x, the HCF becomes 3x. Thus, the factorized form of the expression can be represented as 3x(2 - 3). This method is essential for simplifying expressions and solving equations effectively.
What are perfect squares in algebra?
Perfect squares in algebra are expressions that can be expressed as the square of a binomial. For example, the expression x^2 - 16 is a difference of two perfect squares because both x^2 and 16 are perfect squares (the square of x and the square of 4, respectively). To factor such expressions, you set up two brackets with a minus sign and a plus sign, resulting in (x - 4)(x + 4). Recognizing perfect squares is important in algebra as it allows for easier factorization and simplification of expressions.
How do I factor a difference of squares?
To factor a difference of squares, you need to identify two perfect squares separated by a minus sign. The general formula for this is a^2 - b^2 = (a - b)(a + b). For example, in the expression 9p^2 - 1, you can see that 9p^2 is the square of 3p and 1 is the square of 1. Therefore, you can factor it as (3p - 1)(3p + 1). This method is particularly useful in algebra for simplifying expressions and solving quadratic equations, as it allows for quick factorization.
Why is factorization important in algebra?
Factorization is important in algebra because it simplifies expressions and makes solving equations more manageable. By breaking down complex expressions into their factors, you can identify solutions more easily and understand the relationships between different terms. For instance, when you factor an expression like 121 - 36 into (11 - 6)(11 + 6), it becomes easier to analyze and solve. Additionally, factorization plays a crucial role in polynomial equations, allowing for the identification of roots and simplifying calculations in various mathematical contexts.
Related videos

jawiskunde
Ontbinden in factoren

Khan Academy
Factoring completely with a common factor | Algebra 1 | Khan Academy
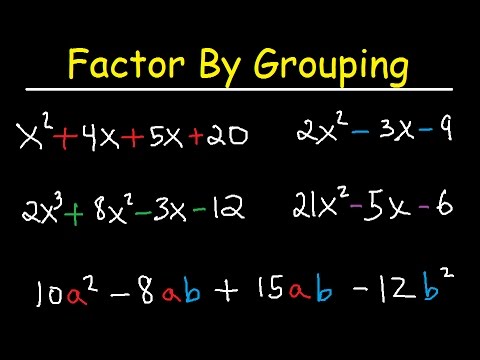
The Organic Chemistry Tutor
Factor By Grouping Polynomials - 4 Terms, Trinomials - 3 Terms, Algebra 2
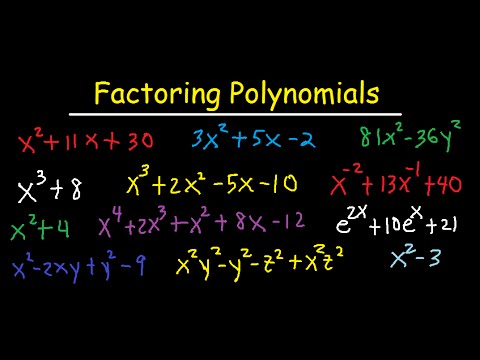
The Organic Chemistry Tutor
Factoring Polynomials - By GCF, AC Method, Grouping, Substitution, Sum & Difference of Cubes

The Organic Chemistry Tutor
How To Factor Polynomials The Easy Way!
Summary
00:00
Understanding Factorization and Perfect Squares
- Factorization is the inverse of simplification or distribution, where simplifying involves distributing a number into brackets, while factorization involves taking out the highest common factor (HCF) from an expression, such as factoring 4x + 8 into 4(x + 2) by dividing each term by 4.
- To find the HCF of two terms, identify the largest number and the most variables that can be factored out; for example, in the terms 6x and -9, the HCF is 3x, resulting in the factorized form 3x(2 - 3).
- When factorizing, if the terms do not share variables, focus on the numerical factors; for instance, in the terms 10x and -5, the HCF is 5, leading to the factorized expression 5(2x - 1).
- In cases where variables are present, take the lowest exponent of the variable; for example, in 2x^4 and -6x^3, the HCF is 2x^3, resulting in the factorized form 2x^3(x - 3).
- The difference of two perfect squares requires a minus sign between two square roots; for example, x^2 - 16 can be factored into (x - 4)(x + 4) by taking the square root of x^2 (which is x) and the square root of 16 (which is 4).
- When applying the difference of two perfect squares, always set up two brackets with a minus and a plus; for instance, for 9p^2 - 1, the factorization is (3p - 1)(3p + 1) by taking the square root of 9p^2 (which is 3p) and the square root of 1 (which is 1).
- If a term is a perfect square, such as 1, remember that it can always be factored; for example, in the expression 9p^2 - 1, the factorization is (3p - 1)(3p + 1) since both terms are perfect squares.
- Always ensure to use two brackets when factorizing a difference of two perfect squares, as seen in the example of 121 - 36, which factors to (11 - 6)(11 + 6) by taking the square roots of 121 (11) and 36 (6).




