Introduction to point-slope form | Algebra I | Khan Academy
Khan Academy・5 minutes read
The equation of a line can be derived from its slope and a point on the line, resulting in the point-slope form \( y - b = m(x - a) \), which can be further converted into slope-intercept form. Both forms, \( y - b = m(x - a) \) and \( y = mx + b \), serve distinct mathematical purposes by clearly indicating the slope and y-intercept.
Insights
- The slope of a line, defined as the change in y over the change in x, can be used alongside a specific point on the line to derive its equation in point-slope form, which is represented as \( y b = m(x - a) \). This form highlights both the slope and the coordinates of the point, making it a practical tool for understanding linear relationships.
- To transition from point-slope form to slope-intercept form, one can distribute the slope and simplify the equation, ultimately revealing the y-intercept. For example, starting with a slope of 2 and a point (-7, 5), the equation can be transformed to \( y = 2x + 19 \), illustrating how both forms serve distinct purposes in mathematics by clearly indicating the slope and y-intercept.
Get key ideas from YouTube videos. It’s free
Recent questions
What is the slope of a line?
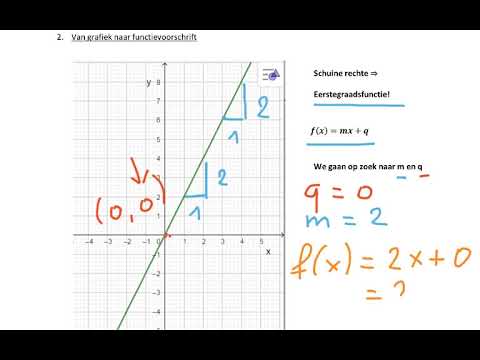
The slope of a line is a measure of its steepness, defined mathematically as the ratio of the vertical change (rise) to the horizontal change (run) between two points on the line. It is often represented by the letter "m" in equations. A positive slope indicates that the line rises as it moves from left to right, while a negative slope indicates that it falls. The slope can be calculated using the formula \( m = \frac{y_2 - y_1}{x_2 - x_1} \), where \( (x_1, y_1) \) and \( (x_2, y_2) \) are coordinates of two distinct points on the line. Understanding the slope is crucial in various applications, including graphing linear equations and analyzing relationships between variables.
How do you find a line's equation?
To find the equation of a line, you typically need the slope and a point through which the line passes. The most common method is to use the point-slope form of the equation, which is expressed as \( y - b = m(x - a) \), where \( m \) is the slope and \( (a, b) \) is the point on the line. If you have the slope and a specific point, you can substitute these values into the equation to derive the line's equation. Additionally, if you need the slope-intercept form, you can manipulate the point-slope form by distributing the slope and rearranging the equation to isolate \( y \). This process allows you to express the line in a format that clearly shows both the slope and the y-intercept.
What is point-slope form?
Point-slope form is a way to express the equation of a line using its slope and a specific point on the line. The general formula is \( y - b = m(x - a) \), where \( m \) represents the slope, and \( (a, b) \) is the coordinate of the point. This form is particularly useful when you know the slope of the line and a point through which it passes, as it allows for easy construction of the line's equation. By using point-slope form, you can quickly visualize how the line behaves relative to the given point and slope, making it a valuable tool in algebra and geometry.
What is slope-intercept form?
Slope-intercept form is a way to express the equation of a line that highlights both the slope and the y-intercept. The standard format is \( y = mx + b \), where \( m \) is the slope of the line and \( b \) is the y-intercept, the point where the line crosses the y-axis. This form is particularly useful for graphing linear equations, as it allows you to easily identify the slope and the starting point on the y-axis. To convert from point-slope form to slope-intercept form, you can distribute the slope and rearrange the equation, which provides a clear representation of the line's characteristics and makes it easier to analyze its behavior.
Why are line equations important?
Line equations are fundamental in mathematics and various applications because they provide a way to model relationships between variables. They are essential in fields such as physics, economics, and engineering, where linear relationships often occur. By using line equations, one can predict outcomes, analyze trends, and solve problems involving linear relationships. Understanding how to derive and manipulate these equations allows for effective communication of mathematical concepts and facilitates the exploration of more complex functions. Additionally, line equations serve as the foundation for more advanced topics in algebra and calculus, making them a crucial component of mathematical education.
Related videos
Summary
00:00
Understanding Line Equations and Their Forms
- The equation of a line can be derived using its slope (m) and a point (a, b) on the line, where the slope is defined as the change in y over the change in x, represented mathematically as \( m = \frac{y - b}{x - a} \).
- To simplify the equation, multiply both sides by \( x - a \) to eliminate the denominator, resulting in the point-slope form of the equation: \( y - b = m(x - a) \), which clearly shows the slope and the specific point on the line.
- For a practical example, if the slope is 2 and the line passes through the point (-7, 5), the point-slope form can be expressed as \( y - 5 = 2(x + 7) \), which directly incorporates the slope and the coordinates of the point.
- To convert the point-slope form to slope-intercept form, distribute the slope (2) and simplify: \( y - 5 = 2x + 14 \), then add 5 to both sides to yield \( y = 2x + 19 \), clearly indicating the slope and y-intercept.
- The two forms of the line's equation, point-slope form \( y - b = m(x - a) \) and slope-intercept form \( y = mx + b \), are useful for different applications in mathematics, allowing for easy identification of the slope and y-intercept.