How To Factor Polynomials The Easy Way!
The Organic Chemistry Tutor・10 minutes read
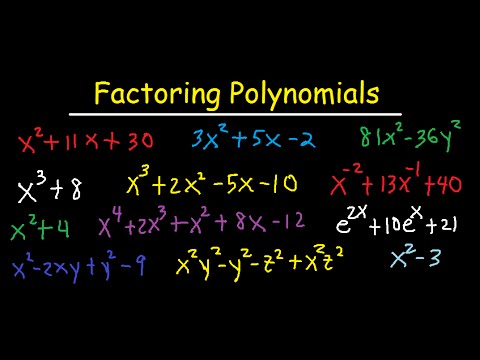
The text provides various strategies for factoring algebraic expressions, including identifying and extracting the greatest common factor (GCF) and factoring trinomials. Each example illustrates the method used, such as factoring out constants and applying grouping techniques to achieve the factored form.
Insights
- To simplify algebraic expressions, it is essential to identify and extract the greatest common factor (GCF), as demonstrated in examples like \(6x 12\) and \(3x^3 - 9x^2\), where factoring out the GCF leads to more manageable forms of the expressions.
- Factoring trinomials, such as \(x^2 + 2x 15\), involves finding two numbers that both multiply to the constant term and add to the linear coefficient, illustrating a systematic approach to breaking down more complex polynomial expressions into simpler binomial factors.
Get key ideas from YouTube videos. It’s free
Recent questions
What is the greatest common factor?
The greatest common factor (GCF) is the largest positive integer that divides each of the numbers in a set without leaving a remainder. It is a fundamental concept in mathematics, particularly in number theory and algebra, as it helps simplify fractions and factor expressions. To find the GCF, one can list the factors of each number and identify the largest one they share. Alternatively, methods such as prime factorization or the Euclidean algorithm can be employed. Understanding the GCF is essential for tasks like simplifying algebraic expressions, solving equations, and performing operations with polynomials.
How do you factor a polynomial?
Factoring a polynomial involves rewriting it as a product of simpler polynomials or expressions. The process typically starts by identifying the greatest common factor (GCF) of the terms in the polynomial, which can be factored out. For example, in a polynomial like \(6x - 12\), the GCF is 6, leading to the factored form \(6(x - 2)\). If the polynomial is a trinomial, one can look for two numbers that multiply to the constant term and add to the coefficient of the linear term. This method is particularly useful for quadratics. Factoring is a crucial skill in algebra, as it simplifies expressions and aids in solving equations.
What is a trinomial?
A trinomial is a polynomial that consists of three terms, typically expressed in the form \(ax^2 + bx + c\), where \(a\), \(b\), and \(c\) are constants, and \(x\) is a variable. Trinomials are often encountered in algebra, especially when dealing with quadratic equations. They can be factored into the product of two binomials, which involves finding two numbers that multiply to the constant term and add to the coefficient of the linear term. For instance, the trinomial \(x^2 + 2x - 15\ can be factored into \((x + 5)(x - 3)\). Understanding trinomials and their properties is essential for solving quadratic equations and graphing parabolas.
What is polynomial grouping?
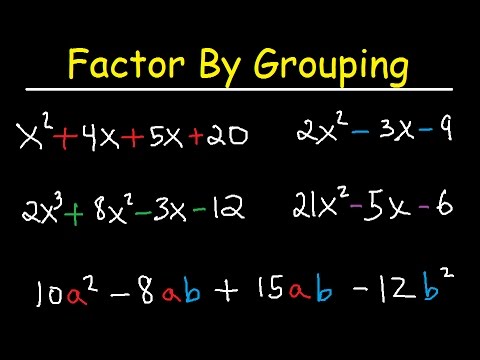
Polynomial grouping is a factoring technique used to simplify polynomials by rearranging and grouping terms in pairs or sets. This method is particularly useful for polynomials with four or more terms. The process involves identifying common factors within the grouped terms, allowing for the extraction of these factors. For example, in the polynomial \(3x^3 - 2x^2 - 12x + 8\), one can group the first two terms and the last two terms, leading to a factored form of \((x^2 - 4)(3x - 2)\). Recognizing patterns, such as the difference of squares, can further aid in the factoring process. Polynomial grouping is a valuable strategy for simplifying complex expressions and solving equations.
How do you simplify an expression?
Simplifying an expression involves reducing it to its most basic form while maintaining its value. This process can include combining like terms, factoring, and eliminating any unnecessary components. For instance, in the expression \(3x^2 - 18x + 24\), one can first factor out the greatest common factor of 3, resulting in \(3(x^2 - 6x + 8)\). Further simplification may involve factoring the quadratic trinomial into \((x - 4)(x - 2)\). Simplifying expressions is a fundamental skill in algebra, as it makes calculations easier and helps in solving equations more efficiently. Understanding how to simplify expressions is crucial for success in higher-level mathematics.
Related videos
The Organic Chemistry Tutor
Factor By Grouping Polynomials - 4 Terms, Trinomials - 3 Terms, Algebra 2

Khan Academy
Factoring completely with a common factor | Algebra 1 | Khan Academy
The Organic Chemistry Tutor
Factoring Polynomials - By GCF, AC Method, Grouping, Substitution, Sum & Difference of Cubes
MathsOnline
Year 9 and 10 - Solving Linear Equations

Mrs Townsend Mathematics - South Africa
Grade 9 Mathematics - Factorisation Part 1
Summary
00:00
Factoring Expressions Using Greatest Common Factors
- To factor the expression \(6x - 12\), extract the greatest common factor (GCF) of 6, resulting in \(6(x - 2)\).
- For \(3x^3 - 9x^2\), the GCF is \(3x^2\), yielding \(3x^2(x - 3)\) after dividing each term.
- In \(4x^2 - 12x\), factor out the GCF of 4 and \(x\), resulting in \(4x(x - 3)\).
- To factor the trinomial \(x^2 + 2x - 15\), find numbers that multiply to -15 and add to 2, giving \(x + 5\) and \(x - 3\).
- For \(2x^2 - 6x - 56\), first factor out 2, leading to \(2(x^2 - 3x - 28)\), then factor to \(2(x - 7)(x + 4)\).
- The expression \(3x^2 - 18x + 24\) factors to \(3(x - 4)(x - 2)\) after extracting the GCF of 3 and factoring the trinomial.
- For \(3x^3 - 2x^2 - 12x + 8\), use grouping to factor as \((x^2 - 4)(3x - 2)\), recognizing \(x^2 - 4\) as a difference of squares.




