U Substitution with Bounds
Dan Schwanekamp・3 minutes read
Perform a u substitution with x^2 + 12 to find the derivative, then calculate the integral from 16 to 48 by simplifying the expression and evaluating the final answer as the square root of 48 minus four.
Insights
- Utilize u substitution when dealing with functions inside radicals to simplify integration and avoid errors, emphasizing the importance of carefully writing integrals.
- Perform anti-derivatives by adjusting exponents and applying appropriate multiplication and division operations, leading to a precise final answer after evaluating the expression within the specified bounds.
Get key ideas from YouTube videos. It’s free
Recent questions
How do you perform u substitution in calculus?
To perform u substitution in calculus, identify a function inside a radical that can be represented as u. Find the derivative of u, solve for DX, and utilize the bounds of the integral. Plug in the bounds to get upper bounds, simplify the expression, and write integrals to avoid errors.
What is the process for finding the anti-derivative?
To find the anti-derivative, add one to the exponent of the function, resulting in U to the power of 1/2. Divide by the exponent and multiply by two over one. Evaluate the expression using the given bounds, cancel out any unnecessary terms, and arrive at the final answer.
Why is it important to write integrals correctly?
It is crucial to write integrals correctly to avoid errors in calculations. By accurately representing the integral, you ensure that the process of solving for the anti-derivative and evaluating the expression is done correctly, leading to the accurate final answer.
How do you simplify expressions in calculus?
To simplify expressions in calculus, identify terms that can be combined or canceled out. Follow the rules of algebra to simplify the expression, ensuring that each step is accurately performed to avoid mistakes in the final result.
What is the significance of bounds in calculus problems?
Bounds in calculus problems define the range over which the integral is evaluated. By utilizing the given bounds, you can determine the upper and lower limits of the integral, allowing for the accurate calculation of the anti-derivative and final answer.
Related videos
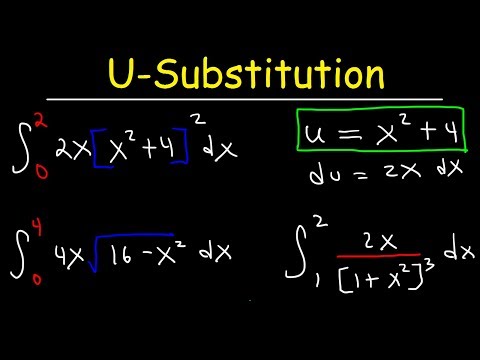
The Organic Chemistry Tutor
U-substitution With Definite Integrals
The Organic Chemistry Tutor
Indefinite Integral - Basic Integration Rules, Problems, Formulas, Trig Functions, Calculus

Professor Leonard
Calculus 1 Lecture 5.2: Volume of Solids By Disks and Washers Method

MathemaTrick
Quadratische Gleichungen lösen – einfach erklärt

The Organic Chemistry Tutor
Implicit Differentiation
Summary
00:00
Integral Calculation with U Substitution and Bounds
- Identify the need for a u substitution due to the function inside the radical, with x^2 + 12 as the u, leading to the derivative being 2x and solving for DX. Utilize bounds of 6 and 2, plugging them in to get upper bounds of 48 and 16, respectively, then proceed with the integral from 16 to 48, simplifying the expression and emphasizing the importance of writing integrals to avoid errors.
- Perform the anti-derivative by adding one to the exponent, resulting in U to the power of 1/2, then dividing by the exponent and multiplying by two over one. Evaluate the expression from 16 to 48, canceling out the 1/2 and two over one, leading to the final answer of the square root of 48 minus four.




