Calculus Made EASY! Learning Calculus
Dr Ji Tutoring・13 minutes read
Calculus focuses on continuous change through differential and integral methods, with differential calculus determining instantaneous rates of change and integral calculus calculating areas under curves. The fundamental theorem of calculus links these two branches by defining them as inverse operations, enhancing problem-solving abilities across numerous fields such as physics and economics.
Insights
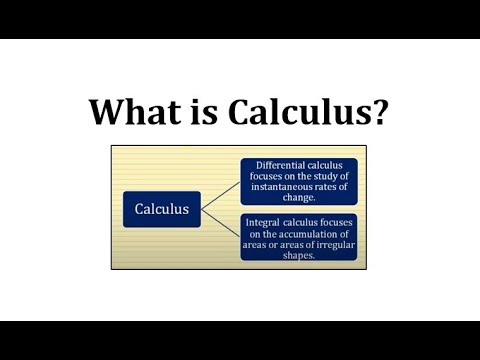
- Calculus is divided into two main branches: differential calculus, which focuses on finding the slope or rate of change at a specific point on a curve, and integral calculus, which calculates the area under curves and volumes of irregular shapes. These branches are interconnected through the fundamental theorem of calculus, which highlights their inverse relationship, where differentiation gives the slope and integration provides the accumulated area.
- The concept of limits is crucial in calculus, as it allows mathematicians to analyze values that approach a certain point, facilitating the precise calculation of slopes in differential calculus and the approximation of areas in integral calculus by summing infinitely small quantities, thereby enhancing problem-solving capabilities across various scientific fields.
Get key ideas from YouTube videos. It’s free
Recent questions
What is calculus in simple terms?
Calculus is a branch of mathematics that studies how things change. It focuses on understanding continuous change, which is different from static mathematics that deals with fixed values. Calculus helps us analyze and model dynamic systems, making it essential in various fields like physics, biology, and economics. By using calculus, we can solve problems related to rates of change and areas under curves, providing valuable insights into how different variables interact over time.
How do you find the slope of a curve?
To find the slope of a curve at a specific point, we use differential calculus, which involves examining two points on the curve that are very close together. By calculating the difference in their y-values divided by the difference in their x-values, we can determine the slope. As the two points get infinitely close, we apply the concept of limits, allowing us to find the instantaneous rate of change at that point. This process gives us a precise measure of how steep the curve is at any given location.
What is the purpose of integral calculus?
Integral calculus is primarily concerned with finding the area under curves and calculating volumes of irregular shapes. It works by approximating these areas through the summation of infinitely thin rectangles under the curve. As the number of rectangles increases, the approximation becomes more accurate, allowing us to determine the exact area. This technique is crucial for solving problems where traditional geometric formulas do not apply, making integral calculus a powerful tool in various scientific and engineering applications.
What are limits in calculus?
Limits are a fundamental concept in calculus that help us analyze the behavior of functions as they approach a certain point. They allow us to understand how values change and behave near specific inputs, which is essential for defining derivatives and integrals. By using limits, we can evaluate functions that may not be directly computable at certain points, enabling us to explore continuous change and the properties of functions in a more nuanced way. This concept is vital for both differential and integral calculus.
How are differentiation and integration related?
Differentiation and integration are two core operations in calculus that are fundamentally linked by the fundamental theorem of calculus. Differentiation involves finding the derivative of a function, which represents the slope or rate of change at any point. In contrast, integration is about finding the anti-derivative, which accumulates area under the curve. The fundamental theorem states that these two processes are inverses of each other, meaning that integrating a function and then differentiating it will return you to the original function, highlighting their interconnected nature in understanding change and accumulation.
Related videos
Mathispower4u
What is Calculus?

Math The World
What Are Derivatives and How Do They Work? Calculus in Context!

Oxford Mathematics
Multivariable Calculus Lecture 1 - Oxford Mathematics 1st Year Student Lecture

Online Mathematics Tutorial
Lebnitz Rule | Differentiation Under Integral Sign

Daniel Palma
07 Integracion (El Universo Mecanico)
Summary
00:00
Understanding Calculus and Its Applications
- Calculus is a branch of mathematics focused on continuous change, differing from static math, which deals with constant values and linear equations.
- Differential calculus, often taught as Calculus 1, aims to find the instantaneous rate of change or slope at any point on a curve.
- Integral calculus, known as Calculus 2, addresses finding the area under curves and volumes in three dimensions, particularly for irregular shapes.
- The concept of limits is fundamental in calculus, allowing for the analysis of values as they approach a certain point, facilitating dynamic calculations.
- To find the slope at a point on a curve, differential calculus uses two points that can be made infinitely close, applying the limit as h approaches 0.
- Integral calculus approximates areas under curves by summing the areas of infinitely thin rectangles, refining the estimate as the number of rectangles increases.
- The area of a triangle can be calculated using the formula: Area = 0.5 × base × height, applicable for simple shapes.
- The relationship between differential and integral calculus is defined by the fundamental theorem of calculus, stating they are inverse operations.
- Differentiating a function f(x) yields its derivative f'(x), representing the slope, while integrating f(x) gives the anti-derivative F(x), representing accumulated area.
- Understanding calculus enhances problem-solving skills in various fields, including physics, biology, and economics, by providing insights into dynamic systems and changes.




