Solving Simultaneous Equations by Elimination (part 2)

Recent questions

• What is the elimination method in math?

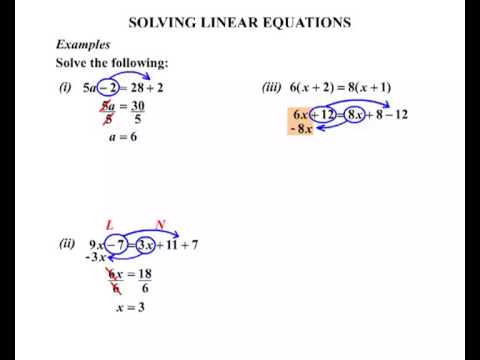
  The elimination method is a technique used to solve systems of equations by eliminating one variable at a time. This is achieved by manipulating the equations to create a situation where adding or subtracting them will cancel out one of the variables, allowing for the other variable to be solved easily. For example, if you have two equations with different coefficients for one variable, you can multiply the equations by suitable numbers to make the coefficients the same. Once the variables are eliminated, you can solve for the remaining variable and then substitute back to find the other variable. This method is particularly useful for solving linear equations and can simplify complex systems into manageable calculations.

• How do I solve a system of equations?

  To solve a system of equations, you can use various methods, including substitution, elimination, or graphing. The elimination method involves aligning the equations so that one variable can be eliminated through addition or subtraction. First, ensure the equations are in standard form, then manipulate them to have the same coefficients for one variable. After eliminating one variable, solve for the remaining variable, and substitute that value back into one of the original equations to find the other variable. This systematic approach allows you to find the values of both variables that satisfy both equations simultaneously.

• What does substituting values mean in math?

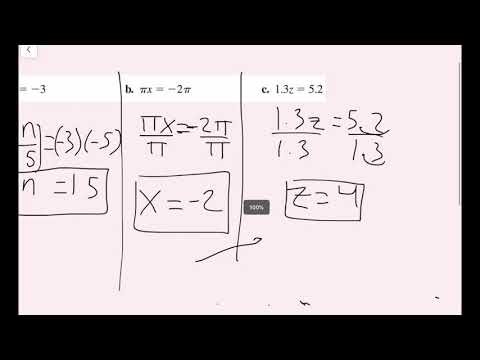
  Substituting values in math refers to the process of replacing a variable in an equation with a specific number or expression. This is often done after solving for one variable in terms of another, allowing you to find the numerical value of the remaining variable. For instance, if you have determined the value of x, you can substitute this value back into one of the original equations to calculate the corresponding value of y. This step is crucial in solving systems of equations, as it helps to verify the solution and ensures that both variables satisfy the original equations.

• Why do we adjust coefficients in equations?

  Adjusting coefficients in equations is necessary when using the elimination method to ensure that the coefficients of one variable are the same across the equations. This allows for the elimination of that variable when the equations are added or subtracted. By multiplying the entire equation by a suitable number, you can create equivalent equations that facilitate the elimination process. This adjustment is essential for simplifying the system of equations, making it easier to solve for the remaining variable. It ensures that the solution is accurate and that both equations are still valid after the manipulation.

• What is the purpose of solving equations?

  The purpose of solving equations is to find the values of the variables that make the equations true. In the context of systems of equations, this means determining the point at which the equations intersect, which represents the solution to the system. Solving equations is fundamental in mathematics as it allows us to model and understand relationships between quantities, make predictions, and solve real-world problems. Whether in algebra, calculus, or applied mathematics, finding solutions to equations is a critical skill that underpins much of mathematical reasoning and analysis.