Go First Dice - Numberphile
Numberphile・15 minutes read
Four friends found a solution to determine the order of play using dice that prevent draws, with unique numbers on each die, allowing for subsets and fairness for different group sizes. Different sets of custom dice were created for five and six players, ensuring every order is equally likely, with ongoing efforts to find even smaller sets while maintaining symmetry, sponsored by Brilliant for those interested in mathematical concepts.
Insights
- The Go First dice, created by Eric Harshbarger and Robert Ford, are designed to determine the order of play among friends without the possibility of draws, featuring unique numbers on each of the four 12-sided dice.
- Michael Purcell's innovative solution involves using a set of 5 120-sided dice, created by replicating 12-sided dice and manipulating permutations, ensuring that every order of play among five players is equally likely, addressing the challenge of fairness in determining game sequences.
Get key ideas from YouTube videos. It’s free
Recent questions
How can dice rolling avoid draws?
By using unique numbers on specialized dice.
How can dice be fair for different group sizes?
By allowing subsets for varied player numbers.
What is the purpose of 120-sided dice?
To ensure every order of play is equally likely.
How can dice biases be balanced?
By creating multiple copies and performing permutations.
What is the goal of finding smaller dice sets?
To minimize total sides while maintaining symmetry.
Related videos

PBS Infinite Series
Higher-Dimensional Tic-Tac-Toe | Infinite Series

singingbanana
A Mathematical Card Trick

A Million Card Tricks
Interactive Flip: This Easy Card Trick Will FOOL Anybody! Performance And Tutorial!
The Organic Chemistry Tutor
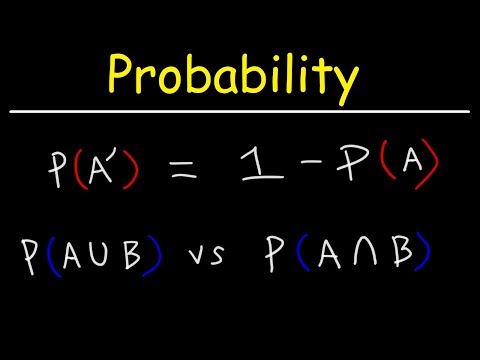
Probability of Complementary Events & Sample Space
MindYourDecisions
I Will Predict Your Number - Math Magic Trick
Summary
00:00
Innovative Dice Solutions Ensure Fair Play
- The problem presented involves four friends trying to determine the order of play using dice.
- Traditional dice rolling can lead to draws, prompting the need for a new solution.
- The Go First dice, invented by Eric Harshbarger and Robert Ford, consist of four 12-sided dice with unique numbers from 1 to 48, ensuring no draws.
- These dice allow for subsets as well, making them equally fair for different group sizes.
- A new solution was sought for five players to ensure every order of play is equally likely.
- A set of 5 60-sided dice was created by the speaker, ensuring equal likelihood of every player's position.
- The speaker collaborated with Brian Pollock to develop this solution through a structured computer search.
- To achieve every order being equally likely, a set of 5 120-sided dice was introduced by Michael Purcell.
- Purcell's method involved creating 10 copies of 12-sided dice and performing permutations on the rows to balance biases.
- This new set of dice ensures every order is equally likely, a concept previously explored by the Polylog YouTube channel.
12:31
Innovative dice solutions for arranging players
- The problem of arranging five people in order can be solved in various ways, such as using cards with numbers or a single 120-sided die, but the fun lies in each player having their own die to roll.
- For six players, a more efficient solution has been found using a set of one 20-sided die and five 360-sided dice, created through a combination of different methods.
- The search for an even smaller set of dice continues, with the goal of finding a set of five 30-sided dice, or potentially five 60-sided dice, to minimize the total number of sides while maintaining symmetry.
- The episode is sponsored by Brilliant, offering interactive lessons and courses related to dice and other mathematical concepts, with a free trial and discount available for those interested in expanding their knowledge.




