Algebra 1 Basics for Beginners
UltimateAlgebra・2 minutes read
To solve equations, follow the steps to isolate the variable by performing opposite operations, handling different types of equations like multi-step or absolute value, solving inequalities, graphing on number lines, and applying the concept to word problems. Functions must have each input value mapping to a single output to be considered a function, as opposed to relations where one input can have multiple outputs.
Insights
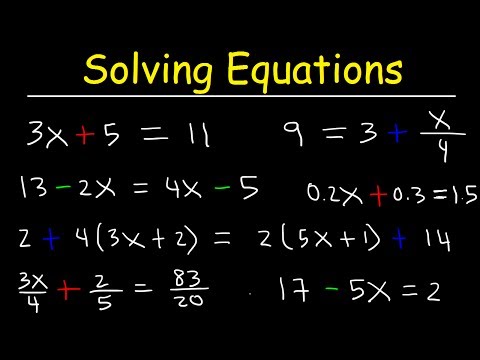
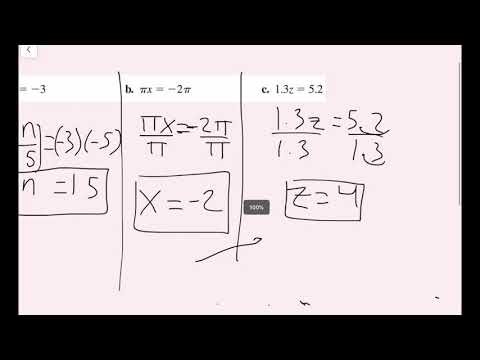
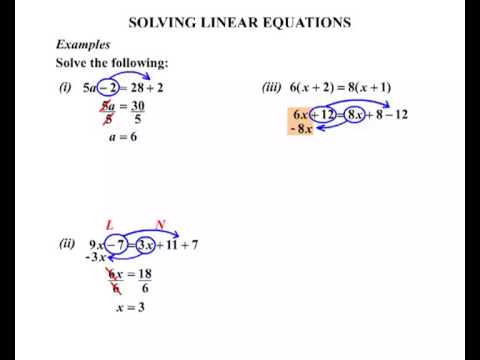
- Solving equations involves performing opposite operations to isolate the variable; for one-step equations, remove terms by performing inverse operations, while multi-step equations require systematically eliminating all attached terms to find the solution.
- When dealing with absolute value equations, consider both positive and negative solutions by solving two separate equations; for radical equations, square both sides to remove the radical term and solve for x.
Get key ideas from YouTube videos. It’s free
Recent questions
How do you solve one-step equations?
By moving variable terms to one side.
What is the process for solving multi-step equations?
Eliminate terms sequentially, then isolate the variable.
How do you solve absolute value equations?
Solve for positive and negative values separately.
What is the approach to solving radical equations?
Isolate the radical term and square both sides.
How do you solve inequalities?
Treat them like equations, reversing signs when needed.
Related videos
The Organic Chemistry Tutor
Algebra - How To Solve Equations Quickly!
Mr. G Math
Solving Simple Equations (1.1 Big Ideas Math - Algebra 1)
The Organic Chemistry Tutor
Algebra Introduction - Basic Overview - Online Crash Course Review Video Tutorial Lessons

Mr. G Math
Solving Equations with Variables on Both Sides (1.3 Big Ideas Math - Algebra 1)
MathsOnline
Year 9 and 10 - Solving Linear Equations
Summary
00:00
Equation Solving Techniques for Various Scenarios
- To solve one-step equations, move all terms with the variable to one side by performing the opposite operation; for example, in x + 2 = 5, subtract 2 from both sides to get x = 3.
- For two-step equations like 2x + 3 = 11, first eliminate addition/subtraction terms, then tackle multiplication/division; subtract 3 from both sides, then divide by 2 to find x = 4.
- Multi-step equations, such as 3x^2 + 8 = 20, require removing all terms attached to the variable; subtract 8, divide by 3, then find the square root to get x = 2.
- When x appears twice in an equation, like 4x + 5 = 9 + 2x, move one set of x terms to one side by subtracting 2x; then, eliminate other terms to find x = 2.
- Absolute value equations, e.g., |x + 3| = 7, involve solving for both positive and negative values; solve two equations, x + 3 = 7 and x + 3 = -7, to find x = 4 and x = -10.
- In equations like |x + 1| + 6 = 9, isolate the absolute value term by removing other terms; solve for x by equating the absolute value to positive and negative values, finding x = -4 or x = 2.
- Radical equations, where x is under a square root, require isolating the radical term; square both sides to eliminate the square root and solve for x.
- Rational equations, like 4 / (x - 5) = 3 / x, involve cross-multiplying to remove fractions; simplify to find x = -5.
- To solve for x in y = mx + b, rearrange the equation by moving terms to isolate x; subtract b, then divide by m to find x = (y - b) / m.
- In inequalities, such as -3x + 1 > 7, treat them like equations but remember to reverse the inequality sign when dividing or multiplying by a negative; solve to find x < -2.
- Combined inequalities, like -3 < x + 8 < 20, require solving each part separately and then combining the solutions; subtract 8 to find -11 < x < 12.
- Graphing inequalities involves plotting points on a number line and shading appropriately; for x > -4, plot -4 with an open circle and draw an arrow to the right.
- In word problems, like packaging 2,500 gallons into 20 boxes with 100 gallons left, identify the total, the group (boxes), and the remaining amount; solve by setting up equations to find the number of gallons in each box.
17:54
Solving Equations and Identifying Functions Efficiently
- To find the value of X, start with the equation 20x = 2,400 by subtracting 100 from both sides, then divide by 20 to get X = 120, indicating there were 120 gallons in each box.
- For faster problem-solving, skip unnecessary steps and directly write the two-step equation, such as 20x + 100 = 2,500, then solve by subtracting 100 and dividing by 20 to get the answer.
- In identifying functions, ensure each input value corresponds to only one output value; a relation is not a function if an input has multiple outputs, as seen in the example where input two has outputs of seven and five, making it not a function.




